For Fri, Sep 24:

• turn in 17.1 - 1,2,9,10,11,13,14 and 17.2 - 1,2
• Also, do and turn in the following: If C₁ and C₂ are real constants, verify that the function $x(t) = C_1 \cos(\omega t) + C_2 \sin (\omega t)$ is a solution of the Hooke's Law spring equation $x''(t)=-\omega^2 x(t)$. Show that this solution is actually a sinusoidal motion $A \sin(\omega t + \phi)$ for some constants A (the amplitude) and $\phi$ (the phase). What are A and $\phi$ in terms of C₁ and C₂? What is the geometric interpretation of A and $\phi$? Draw a careful sketch in the case when C₁ = C₂ = 1, and do the addition graphically to plot the graph of $x(t) = C_1 \cos(\omega t) + C_2 \sin (\omega t)$. Does the sketch agree with your analytic determination of the amplitude and phase in this case?
• read 7.9 (only separable equations) and 17.2.

For Mon, Sep 27:

• turn in 17.1 - 15,17 and 17.2 - 3,6,7,8,14,21 and 6.1 - 2 and 6.2 - 1.
• Do and turn in the following problem: Newton's law of cooling states that, if a hot object of temperature T is exposed to an ambient environment of constant cooler temperature A, then the rate of change of temperature of the object is proportional to the difference between the temperature of the object and the temperature of the ambient environment.
  • Express this relationship as a differential equation. Does it matter whether you use the centigrade or the Kelvin temperature scale? Why or why not?
  • A cross-country skier takes a pot of boiling tea (100 degrees C) outside from the lodge. Five minutes after she leaves the lodge, the tea has cooled to 50 degrees C. Ten minutes after her departure from the lodge, the tea has cooled to 20 degrees C. How cold is it outside?
• read 6.1. Skim 6.2 and 6.3.

For Wed, Sep 29:

• turn in 6.1 - 3,7,8,12 and 6.2 6,8,13 and 17.4 - 1,2
• read 7.9 and 17.4

For Fri, Oct 1:

• turn in 6.1 - 20,23,30, 6.2 - 28,44, 6.3 - 4,11, 17.4 - 3,4,7,8, 17.6 - 1, Appendix I - 1,5

• read 17.6, Appendix I, and the handout on the complex exponential

For Mon, Oct 4:

• turn in 17.6 - 2,3,4, Appendix I - 15,23,31,32,40,53, 3.7 - 2

• read 3.7 and 17.7

For Wed, Oct 6:

• turn in 3.7 - 1,14,18,19,20,21,22,28, 7.1 - 2

• read 3.7 and 17.7 again. Also read 7.1

For Fri, Oct 8:

• turn in 7.1 3,5,6,9,13. Also:

  1.

  Prove that an overdamped or critically damped freely vibrating spring passes through the equilibrium position (the ``relaxed" natural rest position the spring would assume if it were not vibrating) at most once.

  2.

  Consider an oscillatory motion of frequency $\omega$ given by $x(t) = C_1 \cos(\omega t) + C_2 \sin (\omega t)$. Show that x(t) can also be written as $x(t) = Re(Ae^{i\omega t})$ for some complex constant A. (HINT: recall an analagous problem from the first homework handout.)

• read 7.1 again.

For Mon, Oct 11

• turn in 7.1 - 16,17,19,21 and 4.8 - 1,2 and 9.2 - 2

• read sections 4.8 and 9.2

For Wed, Oct 13

• turn in 4.8 - 7,8,13,17,21,27 and 9.2 - 1,4,21 and 9.7 - 2 and 9.8 - 2. Also:

  An object of mass m is thrown upward with initial velocity v₀; the gravitational acceleration is g (directed downward), and there is a retarding force (due to air resistance) which is proportional to the speed. In terms of the constants in the problem (the mass, the gravitational acceleration, the initial velocity, and the constant of proportionality describing the air resistance), determine the ascent time (the time until the object reaches its highest point and begins its descent).

• read section 9.3, beginning with the Ratio Test (p. 542) to the end of the section, section 9.5 through the Radius of Convergence topic (skim the rest), sections 9.7 and 9.8. Note: this appears to be a longer reading assignment than it actually is, since the topics we wish to cover are scattered about in the book. To help you focus on the important points, note that we are mainly concerned with the application of the Ratio Test to determining the radius of convergence of a power series, and with the approximation of functions by Taylor polynomials. We will combine these two ideas in the next reading assignment.

For Fri, Oct 15

• turn in 9.7 - 5,6,10 and 9.8 - 3,4,12,15 and 9.6 - 1

• read sections 9.6 and 9.9

For Mon, Oct 18

• turn in 9.6 - 6,7,9,16,26,27,31,33,34. Note: in the exercises for section 9.6, try to find the Taylor series from known series (sin, cos, exp, the geometric series, etc.) by using the operations on power series we've discussed, not by calculating the coefficients directly.

• read sections 10.1 and 10.2

For Wed, Oct 20

there will be no homework to be turned in.

• read section 10.2 again and read sections 10.3 and 10.4.

For Fri, Oct 22

• turn in 10.1 - 4,9,17,26,31,40 and 10.2 - 3,13,16,22,24 and 10.3 - 1.

• read sections 10.3 and 10.4 again.

For Mon, Oct 25

• turn in 10.2 - 26,30,31 and 10.3 - 3,4,6,14,15,21,22 and 10.4 - 15.

• read section 10.4 again.

For Wed, Oct 27

• turn in 10.3 - 23,25 and 10.4 - 2,4,5,14, and the following problem on determinants:

  Note the set of row vectors in the form
  

  $$v = [ x_1 \cdots x_n ]$$
  
  
  form a n-dimensional vector space when addition is given by addition of the components and cv means multiplying each component by c. It is also useful to let
  
  $$e_i =[ \begin{array}{ccccccc} 0 & \cdots & 0 & 1 & 0 & \cdots & 0 \end{array}]$$
  
  
  where the 1 is in the ith component, and note
  
  $$v = \sum_{i= 1}^n x_i e_i.$$
  
  

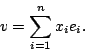
  Similarly, the vector space of n x n matrixes is given by all vectors in the form
  

  $$A = \left[ \begin{array}{c} v_1 \\ \cdot \\ \cdot \\ \cdot \\... ...} & \cdot & \cdot & \cdot & x_{nn} \\ \par\end{array}\right] .$$
  
  

  The determinant, det, can be viewed as a function on matrixes satisfying the following four properties

  1.

  
  

  $$det\left[ \begin{array}{c} v_1 \\ \cdot \\ v_{i+1} \\ v_i \\ ... ...c} v_1 \\ \cdot \\ \cdot \\ \cdot \\ v_n \end{array} \right]$$

  
  

  2.

  
  

  $$det\left[ \begin{array}{c} u_1 + w_1 \\ v_2 \\ \cdot \\ \cdot... ...\ v_2 \\ \cdot \\ \cdot \\ \cdot \\ v_n \end{array} \right]$$

  
  

  3.

  
  

  $$det\left[ \begin{array}{c} c v_1 \\ v_2 \\ \cdot \\ \cdot \\ ... ...\ \cdot \\ \cdot \\ \cdot \\ v_n \end{array} \right] \right)$$

  
  

  4.

  
  

  $$det\left[ \begin{array}{c} e_1 \\ \cdot \\ \cdot \\ \cdot \\ e_n \end{array} \right] = 1.$$

  
  

  If you are confused by the notation describing property one, it is saying if you interchange neighboring rows in the matrix then the determinant's value changes sign. Note that by property 1, properties 2 and 3 hold for any row. It is a fact that the determinannt exist for any n and is uniquely determined by these four properties. In the book you are given the formulas for the 2 x 2 and 3 x 3 determinats, via
  

  $$det \left[ \begin{array}{cc} a & b \\ c & d\\ \end{array}\right] = ad -bc.$$
  
  
  and
  
  $$det\left[ \begin{array}{c} u \\ v \\ w \end{array}\right] = u \cdot (v \times w) .$$
  
  

  Exercises:

  1.

  Check that the above formula for the 2 x 2 determinant satisfies properties 1-4.

  2.

  Check that the above formula for the 3 x 3 determinant satisfies properties 1-4. (Hint: you may want to look at exercise 10.3 - 18 which is immediately true due to the volume interpretation of the scalar triple product).

• read section 10.4 again and read section 10.6.

for Thurs, Oct 28

• turn in 10.4 - 10,11,16,17,18,21,25,27.

• read section 10.6.

for Mon, Nov 1

• turn in 10.4 - 26,28,29,30 from the textbook and 2.2 - 1,2,4,5 and 2.3 - 1,2 from the handout.

• read section 2.3 from the handout.

for Wed, Nov 3

• turn in 2.3 - 3,4,5,6,7,9,11,12 and 3.1 - 1,2,3 from the handout.

• read section 3.1 from the handout.

for Fri, Nov 5

• turn in 2.3 - 8,10,13 and 3.1 - 4,5,6,7,9 and 4.1 - 1 from the handout.

• read section 4.1 from the handout.

for Mon, Nov 8

• turn in 3.1 - 8,10,11,12,13 and 4.1 - 2,3,4,5,6,7 and 4.2 - 1,2,3 from the handout.

• Study for exam.

for Wed, Nov 10 Study for the exam. Do the practice exam, you will not have to turn this in.

Here a few corrections.

1.

Number 4: the second plane should be

A₂ x + B₂ y + C₂ z + D₂ =0,

not

A₁ x + B₁ y + C₁ z + D₁ =0.

2.

Number 8: the point D should be D = (1,-1), not (-1,1).

3.

Number 6: first note that by the set of cubic polynomials it is meant all polynomilas in the form a₃ x³ + a₂ x² + a₁ x + a₀ where the a_i are real numbers. ( i.e. we are allowing that any of the a_i are zero. So for example, for the purposes of this problem a quadradic polynomial is a degenerate example of a cubic polynomia.) Secondly, to do the the problem you are required to chose a range of T there are several possible choices. You may fail to be able to do part (c) if you don't make a finite dimensional choice, so this you should do.

for Fri, Nov 12

• turn in 12.1 - 2,22,25,27,28,29,30 and 12.2 - 4,8,14 and 12.3 - 3

• Read 12.1, 12.2 (if you haven't already), and 12.3 and 12.4

for Mon, Nov 15

• turn in 12.3 - 7,8,13,15,19,23,30 and 12.4 - 3,5,11,12,17,18
• Read the handout.

for Wed, Nov 17

• turn in 12.5 - 2,3,6,8, (see Note) 16,18,19,24,35 (oops I meant 34 so your off the hook dont do either) and 12.6 - 14,15,16. Note: You are required to do problems 2,3,6,8 from the linear transformation point of view to receive full credit. In other words a complete solution will include how to view the function being examined as a composition of mappings, differentiating these mappings, and multiplying out the corresponding derivative matrices in order to find the needed derivative. By two ways you should use the matirx methed as well as constructing the needed function via compostion and differnetiating it.)

• Read 12.7.

for Fri, Nov 19

Note to Prof. Webb's students: on Friday, you are required to turn in the assignment listed above as being due on Wednesday - not the assignment listed below.

• turn in 12.5 - 1,4,7,10,11,12 As above: You are required to do these problems from the linear transformation point of view to receive full credit. 12.5 - 34, and 12.7 - 4,5,9

• Read 13.1

for Mon, Nov 22

• turn in 12.7 - 11,12,17,18,21,22,23,26,28 and 13.1 - 1,3,4

• Read 13.1

for Mon, Nov 29

• turn in 13.1 - 5,6,9,11,14,15,21 and 13.2 - 1,2,4

• Read 13.2

for Wed, Dec 1

• turn in 13.2 - 3,6,7 and 13.3 - 1,3,4,6

• Read 13.3

for Sunday Dec 4 Try this prictice exam (it is of course not due.)

Math 9 Practice Final

1.

(a)

Find the Taylor series about x=0 of

$$\arctan\left(\frac{x}{2}\right).$$

(b)

What is the radius of convergence of this series?

2.

(a)

Find an equation for the tangent plane to the graph of f(x,y) = xy + y² + e^2x at (0,2,5).

(b)

Find an equation for plane parallel to this tangent plane containing the point (1,1,0).

(c)

Where does the line perpendicular to this new plane and containing (1,1,0) hit the original tangent plane.

3.

Let f(x,y) = xy and $g(x,y) = e^y \sin(x)$. Describe the points where the level sets of f and g are perpendicular.

4.

Find the minimum and maximum values of f(x,y) = (x + y)e^{-(x2 + y2)} on the disk $x^2 + y^2 \leq 1$.

5.

Let $f(x,y,z) = ((yz) \sin(xy) , (yz)e^{(xz)} + (xy))$.

(a)

Express f as a composite of a function $g: R^3 \rightarrow R^2$ and a function $f: R^3 \rightarrow R^3$.

(b)

Find Df and Dg (Df refers to the derivative of f).

(c)

Use the chain rule to compute $Df(\frac{\pi}{2},1,1)$.

(d)

Find the kernel of $Df(\frac{\pi}{2},1,1)$.

6.

Notice

$$M_{\lambda} = \left[\begin{array}{rr} \lambda & 0 \\ 0 & 1 \end{array} \right]$$

is a stretch of the plane by $\lambda$ in the direction of the x-axis. Let $M^{\theta}_{\lambda}$ denote the stretch by $\lambda$ in the direction determined by the line inclined at an angle $\theta$ with respect to the x-axis. Find a formula for $M_{\lambda}^{\theta}$.

answer:

$$I + (\lambda -1) \left[\begin{array}{rr} (\cos(\theta))^2 & \... ...(\theta) \sin(\theta) & (\sin(\theta))^2 \end{array} \right] .$$

7.

Let V be the vector space of polynomials of degree $\leq 3$ and let A denote the linear transformation of V to V given by

$$A(p(x)) = a \frac{d^2p}{dx^2} + b \frac{dp }{dx} + c p .$$

(a)

Express A as a matrix.

(b)

In each of the following cases describe the rank of A, and find a non-zero polynomial in A's kernel whenever such an element exists.

i.

$a \neq 0$ and $b \neq 0$ and $c \neq 0$.

ii.

$a \neq 0$ and $b \neq 0$ and c = 0.

iii.

$a \neq 0$ and b = 0 and c = 0.

answer to part (a) if we let x³ corrspond to the first standard basis vector, x² the second, x the third, and 1 the fourth the anser to a is

$$A = \left[\begin{array}{rrrr} c & 0 & 0 & 0 \\ 3 b & c & 0& 0 \\ 6a & 2b & c & 0 \\ 0 & 2a & b & c \end{array} \right] .$$

8.

(a)

Find the general solution to

$$\frac{d^2f}{dx^2} - 3 \frac{df }{dx} + 2 f= 0 .$$

(b)

Use the previous problem (problem 7(a)) to find a polynomial solution to

$$\frac{d^2f}{dx^2} - 3 \frac{df }{dx} + 2 f = x^3 + 3x +1.$$

(c)

Find a solution to

$$\frac{d^2f}{dx^2} - 3 \frac{df }{dx} + 2 f = x^3 + 3x +1$$

satisfying f(0)=1 and $\frac{df}{dx}(0) = 2$.

answers (a)

f(x) = a e^2x + b e^x

(b)

$$p(x) = \frac{1}{8} (4x^3 + 18 x^2 - 54 x -95)$$

(c)

$$f(x) = p(x) - \frac{33}{8}e^{2x} + \frac{136}{3} e^x$$